Grothendieck spectral sequence without spectral sequences

Posted on 12 September 2024 by Remy

Last semester, I taught a course Sheaves in topology, about the cohomology of topological spaces via sheaves. An important and often-used tool is the Grothendieck spectral sequence: if

$\mathscr A \overset F\to \mathscr B\overset G\to \mathscr C$

are left exact functors of abelian categories, with composition $H = GF$ , where $\mathscr A$ and $\mathscr B$ have enough injectives, and $F$ takes injectives to $G$ -acyclic objects, then there is a spectral sequence

$E_2^{i,j} = R^iG(R^jF(A)) \Rightarrow R^{i+j}H(A)$

for any $A \in \mathscr A$ .

However, I do not want to assume spectral sequences, nor spend multiple lectures developing them from scratch. Here is a variant that is easy to prove and often sufficient:

Lemma. Let $\mathscr A \overset F\to \mathscr B\overset G\to \mathscr C$ and $H = GF$ as above, and let $A \in \mathscr A$ .

If $R^iG(R^jF(A)) = 0$ for all $i > 0$ and all $j \geq 0$ , then

$R^iH(A) \cong G(R^iF(A))$

for all $i \geq 0$ .
If $R^iG(R^jF(A)) = 0$ for all $i \geq 0$ and all $j > 0$ , then

$R^iH(A) \cong R^iG(F(A))$

for all $i \geq 0$ .

Proof. For both parts, let $0 \to A \to I \to B \to 0$ be an exact sequence with $I$ injective. The long exact sequences for $R^jF$ and $R^iH$ give

$\arraycolsep=2pt\begin{array}{ccccccccccc} 0 & \to & F(A) & \to & F(I) & \to & F(B) & \to & R^1F(A) & \to & 0,\! \\ 0 & \to & H(A) & \to & H(I) & \to & H(B) & \to & R^1H(A) & \to & 0,\! \end{array}$

and isomorphisms $R^iF(B) \stackrel\sim\to R^{i+1}F(A)$ and $R^iH(B) \stackrel\sim\to R^{i+1}H(A)$ for $i > 0$ . Write $C$ for the image of $F(I) \to F(B)$ , so that we get short exact sequences

(1) $\arraycolsep=2pt\begin{array}{ccccccccc} 0 & \to & F(A) & \to & F(I) & \to & C & \to & 0,\!\\ 0 & \to & C & \to & F(B) & \to & R^1F(A) & \to & 0.\! \end{array}$

Since $F(I)$ is $G$ -acyclic, we get

$0 \to H(A) \to H(I) \to G(C) \to R^1G(F(A)) \to 0$

and isomorphisms $R^iG(C) \stackrel\sim\to R^{i+1}G(F(A))$ for $i > 0$ .

We prove (1) by induction on $i$ . For $i = 0$ , there is nothing to prove. Since $F(A)$ , $F(I)$ , and $R^1F(A)$ are $G$ -acyclic, so are $C$ and $F(B)$ by the short exact sequences of (1), and we get exact sequences

$\arraycolsep=2pt\begin{array}{ccccccccc} 0 & \to & H(A) & \to & H(I) & \to & G(C) & \to & 0,\!\\ 0 & \to & G(C) & \to & H(B) & \to & G(R^1F(A)) & \to & 0.\! \end{array}$

These glue to an exact sequence $0 \to H(A) \to H(I) \to H(B) \to G(R^1F(A)) \to 0$ , which by the exact sequence for $R^iH$ gives $G(R^1F(A)) \cong R^1H(A)$ . This proves the result for $i = 1$ . We also see that $R^jF(B)$ is acyclic for all $j$ : for $j = 0$ , we proved this above, and for $j > 0$ this follows from $R^jF(B) \cong R^{j+1}F(A)$ . Thus $B$ satisfies the same hypotheses as $A$ , and the isomorphisms

$R^iH(A) \cong R^{i-1}H(B) \cong G(R^{i-1}F(B)) \cong G(R^iF(A))$

for $i > 1$ prove the result by induction on $i$ .

Likewise, we prove (2) by induction on $i$ . Again, for $i = 0$ , there is nothing to prove. The long exact sequence of $0 \to C \to F(B) \to R^1F(A) \to 0$ gives $R^iG(C) \cong R^iG(F(B))$ for all $i \geq 0$ since $R^iG(R^1F(A)) = 0$ for all $i \geq 0$ . We obtain an exact sequence

$0 \to H(A) \to H(I) \to H(B) \to R^1G(F(A)) \to 0$

and isomorphisms $R^iG(F(B)) \stackrel\sim\to R^{i+1}G(F(A))$ for $i > 0$ . Comparing with the long exact sequence for $R^iH$ gives $R^1H(A) \cong R^1G(F(A))$ , showing the result for $i = 1$ . Moreover, we have $R^iG(R^jF(B)) = R^iG(R^{j+1}F(A)) = 0$ for all $i \geq 0$ and all $j > 0$ , so $B$ satisfies the same hypotheses as $A$ . Then the isomorphisms

$R^iH(A) \cong R^{i-1}H(B) \cong R^{i-1}G(F(B)) \cong R^iG(F(A))$

show the result for $i > 1$ by induction. $\qedsymbol$

Corollary. If $F$ is exact and takes injectives to $G$ -acyclic objects, then

$R^iH(-) \cong R^iG(F(-)) \colon \mathscr A \to \mathscr C$

for all $i \geq 0$ . Likewise, if $G$ is exact, then the acyclicity assumption on $F(I)$ is automatic, and

$R^iH(-) \cong G(R^iF(-)) \colon \mathscr A \to \mathscr C$

for all $i \geq 0$ . $\qedsymbol$

Example. This is used many times in sheaf cohomology. Some examples:

If $i \colon Z \hookrightarrow X$ is a closed subset, then $H^n(X,i_*\mathscr F) \cong H^n(Z,\mathscr F)$ for all abelian sheaves $\mathscr F$ on $Z$ and all $n \geq 0$ .
If $f \colon W \hookrightarrow X$ is a locally closed subset, then $H^i_c(X,f_!\mathscr F) \cong H^i_c(W,\mathscr F)$ for all abelian sheaves $\mathscr F$ on $W$ .
If $\underline H^i \colon \mathbf{Ab}(X) \to \mathbf{PAb}(X)$ is the derived functor of the inclusion from sheaves into presheaves, then $\underline H^i(\mathscr F)(U) = H^i(U,\mathscr F)$ .
If $\mathscr F$ is a sheaf on $X$ , then the sheafification of $\underline H^i(\mathscr F)$ is $0$ for $i > 0$ .
The higher pushfoward $R^if_*\mathscr F$ along a map $f \colon Y \to X$ is the sheafification of $U \mapsto H^i(f^{-1}(U),\mathscr F)$ .
If $f \colon Y \to X$ is a map and $R^if_*\mathscr F = 0$ for $i > 0$ , then $H^i(Y,\mathscr F) \cong H^i(X,f_*\mathscr F)$ . This is used for the projection $Y \times [0,1] \to Y$ (with $\mathscr F = \underline A$ a constant sheaf) in the proof of homotopy invariance of $H^i(X,\underline A)$ .
If $\mathscr F$ is a sheaf and $\mathscr U = \{U_i \hookrightarrow X\}_{i \in I}$ is an open cover such that $H^i(U_J,\mathscr F) = 0$ for all $i > 0$ and all finite subsets $J \subseteq I$ , then $H^i(X,\mathscr F) \cong \check H^i(\mathscr U,\mathscr F)$ for all $i \geq 0$ . (Here, we write $U_J = \bigcap_{j \in J} U_j$ for $J \subseteq I$ .)
If $X$ is a topological space such that $\mathscr F^\# = 0$ for $\mathscr F \in \mathbf{PAb}(X)$ implies $\check H^i(X,\mathscr F) = 0$ for all $i \geq 0$ , then $H^i(X,\mathscr F) \cong \check H^i(X,\mathscr F)$ for all $\mathscr F \in \mathbf{Ab}(X)$ . (It’s not hard to show that this hypothesis is satisfied if $X$ is paracompact Hausdorff.)

It’s a good exercise to go through all of these and see how they follow from the corollary.

Traces of nilpotent matrices

Posted on 18 November 2022 by Remy

I needed the following well-known result in the course I’m teaching:

Lemma. Let $k$ be a field and $M \in M_n(k)$ a nilpotent matrix. Then $\operatorname{tr} M = 0$ .

The classical proof uses the flag of subspaces

$0 \subseteq \ker M \subseteq \ker M^2 \subseteq \ldots \subseteq \ker M^n = k^n$

to produce a basis for which $M$ is upper triangular. Here is a slick basis-independent commutative algebra proof that shows something better:

Lemma. Let $R$ be a commutative ring with nilradical $\mathfrak r$ , and let $M \in M_n(k)$ be a nilpotent matrix. Then the characteristic polynomial $p_M(t) = \det(tI-M)$ satisfies

$p_M(t) \in t^n + \mathfrak r[t]_{< n}.$

Here we write $I[t]_{<n} \subseteq R$ for the polynomials in $R$ of degree smaller than $n$ whose coefficients lie in a given ideal $I \subseteq R$ .

Note that the formulation should ring a bell: in the previous post we saw that $R[t]^\times = R^\times + t\mathfrak r[t]$ . When $R$ is a domain, this reduces to $R[t]^\times = R^\times$ , and the lemma just says that $p_M(t) = t^n$ .

This suggests that we shouldn’t work with $\det(tI-M)$ but with its anadrome (or reciprocal) $t^n\det(t^{-1}I-M) = \det(I-tM)$ .

Proof of Lemma. We have to show that $\det(I-tM) \in 1 + t\mathfrak r[t]$ . Since $M$ is nilpotent, there exists $r \in \mathbf N$ with $M^{r+1} = 0$ , so $(I-tM)(I+tM+\ldots+t^rM^r) = I$ . Thus $\det(I-tM) \in R[t]^\times = R^\times + t\mathfrak r[t]$ . Evaluating at $t=0$ shows that the constant coefficient is 1. $\qedsymbol$

Invertible polynomials

Posted on 11 November 2022 by Remy

Here is a classic little lemma on polynomials:

Lemma. Let $R$ be a commutative ring with nilradical $\mathfrak r$ . Then $R[t]^\times = R^\times + t\mathfrak r[t]$ .

That is, a polynomial $a_nt^n + \ldots + a_1t + a_0 \in R[t]$ is invertible if and only if $a_0$ is invertible and $a_1,\ldots,a_n$ are nilpotent. This is the formulation you find in Atiyah–MacDonald, exercise 1.2(i). Note that when $R$ is a domain (or more generally a reduced ring), this just says that $R[t]^\times = R^\times$ (via the constant polynomials).

Proof. First suppose $f = \sum_{i=0}^n a_nt^n$ with $a_0 \in R^\times$ and $a_1,\ldots,a_n \in \mathfrak r$ . Multiplying by $a_0^{-1}$ we may assume $a_0 = 1$ ; this does not change the nilpotence assumption on $a_1,\ldots,a_n$ . Then $g := 1-f = -a_1t-\ldots - a_nt^n$ is nilpotent since it is a sum of nilpotent elements $-a_it^i$ in the commutative ring $R[t]$ . Thus we get $g^{r+1} = 0$ for some $r \in \mathbf N$ , and

$(1-g)(1+g+\ldots+g^r) = 1$

shows that $f \in R[t]^\times$ . Conversely, let $f \in R[t]^\times$ , and suppose $fg = 1$ for some polynomial $g = b_mt^m + \ldots + b_1t+b_0 \in R[t]$ . Without loss of generality we may assume $a_n \neq 0 \neq b_m$ . If $R$ is a domain, then the leading term of $fg$ is $a_nb_mt^{m+n} \neq 0$ , so we must have $n=m=0$ and $f$ and $g$ are constant. In the general case, we conclude that $\phi(f) \in S^\times \subseteq S[t]$ for any ring homomorphism $\phi \colon R \to S$ to a domain $S$ . In particular, taking $S = R/\mathfrak p$ for any prime ideal $\mathfrak p \subseteq R$ shows $a_1,\ldots,a_n \in \mathfrak p$ and $a_0 \not\in\mathfrak p$ . Taking the intersection over all prime ideals gives

$\qedsymbol$ $\begin{align*}a_1,\ldots,a_n &\in \bigcap_{\mathfrak p \text{ prime}} \mathfrak p = \mathfrak r,\\ a_0 &\in \bigcap_{\mathfrak p \text{ prime}} (R \setminus \mathfrak p) = R^\times. \end{align*}$

The 14 closure operations

Posted on 6 November 2022 by Remy

I’ve been getting complaints that my lemmas have not been so lovely (or little) lately, so let’s do something a bit more down to earth. This is a story I learned from the book Counterexamples in topology by Steen and Seebach [SS].

A topological space $X$ comes equipped with various operations on its power set $\mathcal P(X)$ . For instance, there are the maps $S \mapsto S^\circ$ (interior), $S \mapsto \bar S$ (closure), and $S \mapsto S^{\text{c}}$ (complement). These interact with each other in nontrivial ways; for instance $(\bar S)^{\text{c}} = (S^{\text{c}})^\circ$ .

Consider the monoid $M$ generated by the symbols $a$ (interior), $b$ (closure), and $c$ (complement), where two words in $a$ , $b$ , and $c$ are identified if they induce the same action on subsets of an arbitrary topological space $X$ .

Lemma. The monoid $M$ has 14 elements, and is the monoid given by generators and relations

$M = \left\langle a,b,c\ \left|\ \begin{array}{cc} a^2=a, & (ab)^2=ab, \\ c^2=1, & cac=b.\end{array}\right.\right\rangle.$

Proof. The relations $a^2 = a$ , $c^2=1$ , and $cac=b$ are clear, and conjugating the first by $c$ shows that $b^2=b$ is already implied by these. Note also that $a$ and $b$ are monotone, and $a(T) \subseteq T \subseteq b(T)$ for all $T \subseteq X$ . A straightforward induction shows that if $v$ and $w$ are words in $a$ and $b$ , then $vaw(S) \subseteq vw(S) \subseteq vbw(S)$ . We conclude that

$abab(S) \subseteq abb(S) = ab(S) = aab(S) \subseteq abab(S),$

so $(ab)^2=ab$ (this is the well-known fact that $ab(S)$ is a regular open set). Conjugating by $c$ also gives the relation $(ba)^2 = ba$ (saying that $ba(S)$ is a regular closed set).

Thus, in any reduced word in $M$ , no two consecutive letters agree because of the relations $a^2=a$ , $b^2=b$ , and $c^2=1$ . Moreover, we may assume all occurrences of $c$ are at the start of the word, using $ac=cb$ and $bc=ca$ . In particular, there is at most one $c$ in the word, and removing that if necessary gives a word containing only $a$ and $b$ . But reduced words in $a$ and $b$ have length at most 3, as the letters have to alternate and no string $abab$ or $baba$ can occur. We conclude that $M$ is covered by the 14 elements

$\begin{array}{ccccccc}1, & a, & b, & ab, & ba, & aba, & bab,\\c, & ca, & cb, & cab, & cba, & caba, & cbab. \end{array}$

To show that all 14 differ, one has to construct, for any $i\neq j$ in the list above, a set $S_{i,j} \subseteq X_{i,j}$ in some topological space $X_{i,j}$ such that $i(S_{i,j}) \neq j(S_{i,j})$ . In fact we will construct a single set $S \subseteq X$ in some topological space $X$ where all 14 sets $i(S)$ differ.

Call the sets $i(S)$ for $i \in \langle a,b\rangle$ the noncomplementary sets obtained from $S$ , and their complements the complementary sets. By the arguments above, the noncomplementary sets always satisfy the following inclusions:
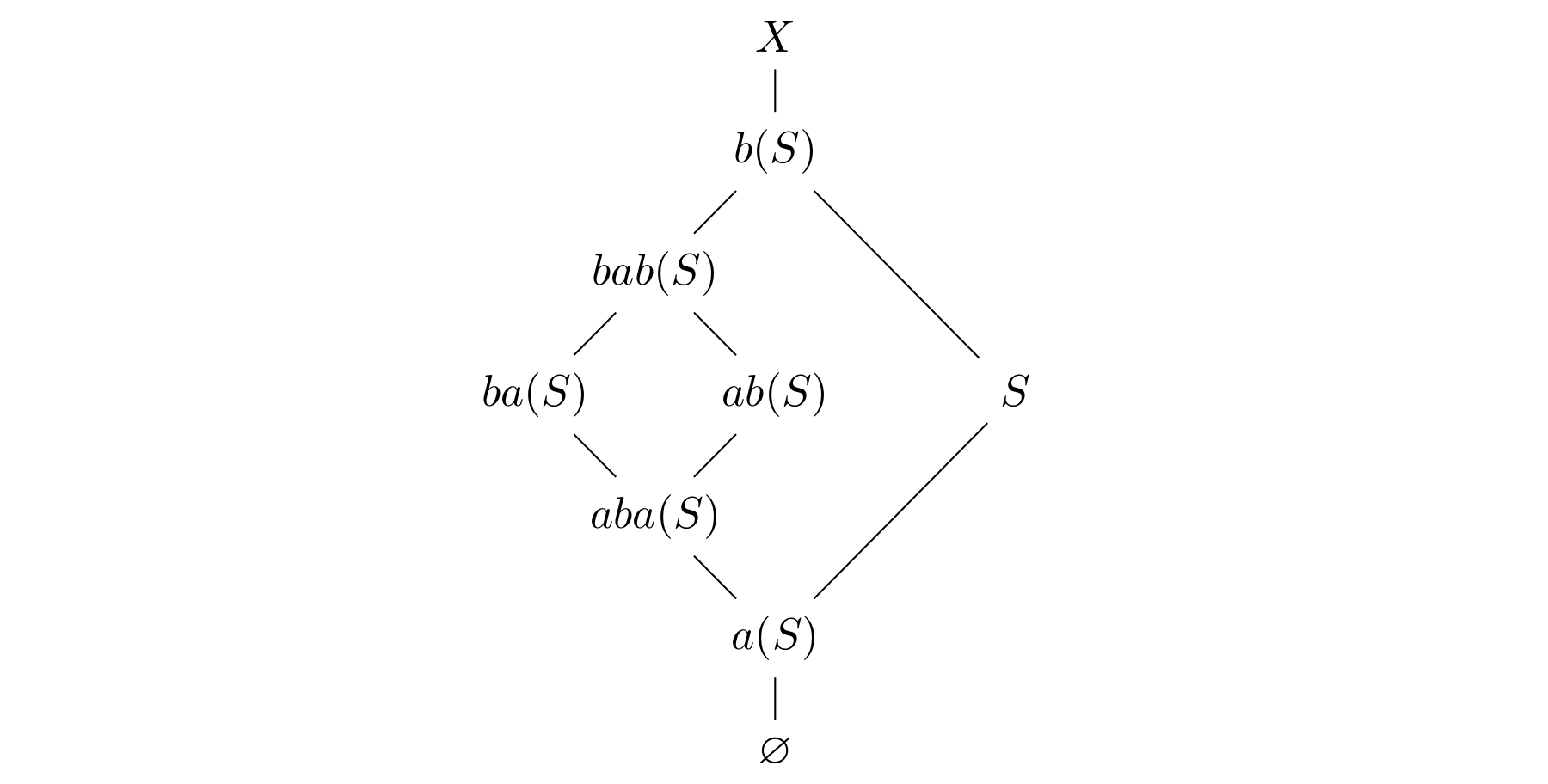It suffices to show that the noncomplementary sets are pairwise distinct: this forces $a(S) \neq \varnothing$ (otherwise $a(S) = ba(S)$ ), so each noncomplementary set contains $a(S)$ and therefore cannot agree with a complementary set.

For our counterexample, consider the 5 element poset $P$ given by

$\begin{array}{ccccc}p\!\!\! & & & & \!\!\!q \\ \uparrow\!\!\! & & & & \!\!\!\uparrow \\ r\!\!\! & & & & \!\!\!s \\ & \!\!\!\nwarrow\!\!\! & & \!\!\!\nearrow\!\!\! & \\ & & \!\!\!t,\!\!\!\!\! & & \end{array}$

and let $X$ be the disjoint union of $P^{\operatorname{Alex}}$ (the Alexandroff topology on $P$ , see the previous post) with a two-point indiscrete space $\{u,v\}$ . Recall that the open sets in $P^{\operatorname{Alex}}$ are the upwards closed ones and the closed sets the downward closed ones. Let $S = \{p,s,u\}$ . Then the diagram of inclusions becomes 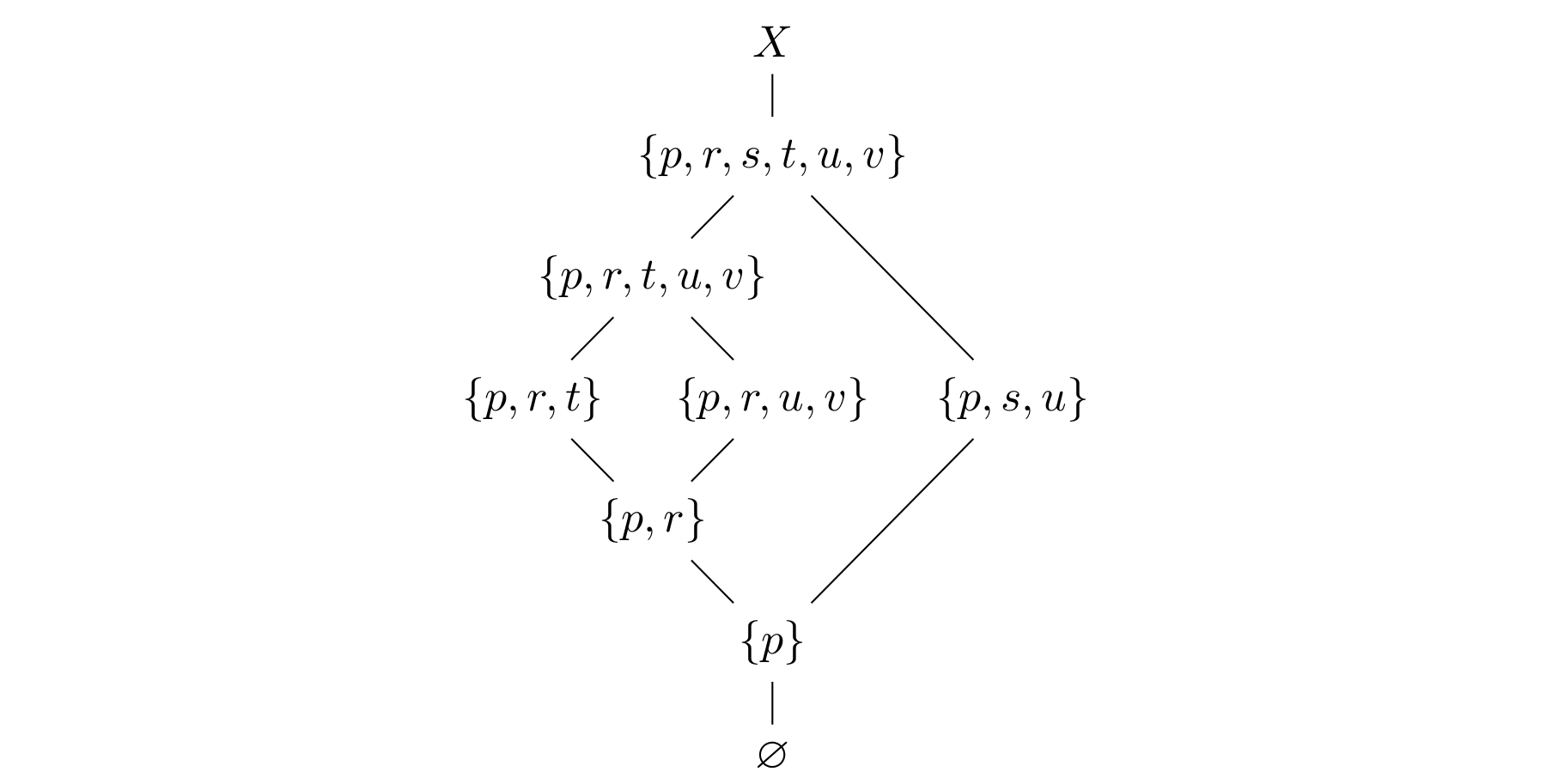We see that all 7 noncomplementary sets defined by $S$ are pairwise distinct. $\qedsymbol$

Remark. Steen and Seebach [SS, Example 32(9)] give a different example where all 14 differ, namely a suitable subset $S \subseteq \mathbf R$ . That is probably a more familiar type of example than the one I gave above.

On the other hand, my example is minimal: the diagram of inclusions above shows that for all inclusions to be strict, the space $X$ needs at least 6 points. We claim that 6 is not possible either:

Lemma. Let $X$ be a finite $T_0$ topological space, and $S \subseteq X$ any subset. Then $aba(S) = ab(S)$ and $bab(S) = ba(S)$ .

But in a 6-element counterexample $X$ , the diagram of inclusions shows that any point occurs as the difference $i(S) \setminus j(S)$ for some $i,j \in \langle a,b\rangle\setminus 1$ . Since each of $i(S)$ and $j(S)$ is either open or closed, we see that the naive constructible topology on $X$ is discrete, so $X$ is $T_0$ by the remark from the previous post. So our lemma shows that $X$ cannot be a counterexample.

Proof of Lemma. We will show that $ab(S) \subseteq aba(S)$ ; the reverse implication was already shown, and the result for $bab(S)$ follows by replacing $S$ with its complement.

In the previous post, we saw that $X$ is the Alexandroff topology on some finite poset $(X,\leq)$ . If $T \subseteq X$ is any nonempty subset, it contains a maximal element $t$ , and since $X$ is a poset this means $u \geq t \Rightarrow u=t$ for all $u \in T$ . (In a preorder, you would only get $u \geq t \Rightarrow u \leq t$ .)

The closure of a subset $T \subseteq X$ is the lower set $\bigcup_{t \in T} X_{\leq t}$ , and the interior is the upper set $\{t \in T\ |\ X_{\geq t} \subseteq T\}$ . So we need to show that if $x \in ab(S)$ and $y \geq x$ , then $y \in ba(S)$ .

By definition of $ab(S)$ , we get $y \in b(S)$ , i.e. there exists $z \in S$ with $z \geq y$ . Choose a maximal $z$ with this property; then we claim that $z$ is a maximal element in $X$ . Indeed, if $u \geq z$ , then $u \geq x$ so $u \in b(S)$ , meaning that there exists $v \in S$ with $v \geq u$ . Then $v \geq z \geq y$ and $v \in S$ , which by definition of $z$ means $v=z$ . Thus $z$ is maximal in $X$ , hence $z \in a(S)$ since $z \in S$ and $X_{\geq z} = \{z\}$ . From $z \geq y$ we conclude that $y \in ba(S)$ , finishing the proof. $\qedsymbol$

The lemma fails for non- $T_0$ spaces, as we saw in the example above. More succinctly, if $X = \{x,y\}$ is indiscrete and $S = \{x\}$ , then $aba(S) = \varnothing$ and $ab(S) = X$ . The problem is that $x$ is maximal, but $X_{\geq x} \supsetneq \{x\}$ and $x \not\in a(S)$ .

References.

[SS] L.A. Steen, J.A. Seebach, Counterexamples in Topology. Reprint of the second (1978) edition. Dover Publications, Inc., Mineola, NY, 1995.

Finite topological spaces

Posted on 1 May 2022 by Remy

One of my favourite bits of point set topology is messing around with easy topological spaces. What could be easier than finite topological spaces? The main result (below) is that the category of finite topological spaces is equivalent to the category of finite preorders.

Recall (e.g. from algebraic geometry) the following definition:

Definition. Let $X$ be a topological space. Then the specialisation preorder on (the underlying set of) $X$ is the relation $x \leq y$ if and only if $x \in \overline{\{y\}}$ .

Note that it is indeed a preorder: clearly $x \leq x$ , and if $x \leq y$ and $y \leq z$ , then $\{y\} \subseteq \overline{\{z\}}$ , so $x \in \overline{\{y\}} \subseteq \overline{\{z\}}$ , showing $x \leq z$ . We denote this preorder by $X^{\operatorname{sp}}$ .

Note that the relation $x \leq y$ is usually denoted $y \rightsquigarrow x$ in algebraic geometry, which is pronounced “ $y$ specialises to $x$ ”.

Definition. Given a preorder $(X,\leq)$ , the Alexandroff topology on $X$ is the topology whose opens $U \subseteq X$ are the cosieves, i.e. the upwards closed sets (meaning $x \in U$ and $x \leq y$ implies $y \in U$ ).

To see that this defines a topology, note that an arbitrary (possibly empty) union or intersection of cosieves is a cosieve. A subbase for the topology is given by the principal cosieves $X_{\geq x} = \{y \in X\ |\ y \geq x\}$ for any $x \in X$ . We denote the set $X$ with its Alexandroff topology by $X^{\operatorname{Alex}}$ .

Likewise, the closed sets in $X$ are the sieves (or downwards closed sets); for instance the principal sieves $X_{\leq x} = \{y \in X\ |\ y \leq x\}$ . The closure of $S \subseteq X$ is the sieve $X_{\leq S} = \bigcup_{s \in S} X_{\leq s}$ generated by $S$ ; for instance the closure of a singleton $\{x\}$ is the principal sieve $X_{\leq x}$ .

Theorem. Let $F \colon \mathbf{PreOrd} \to \mathbf{Top}$ be the functor $X \mapsto X^{\operatorname{Alex}}$ , and $G \colon \mathbf{Top} \to \mathbf{PreOrd}$ the functor $Y \mapsto Y^{\operatorname{sp}}$ .

Let $(X,\leq)$ be a preorder, $Y$ a topological space, and $f \colon X \to Y$ a function. Then $f$ is a monotone function $X \to Y^{\operatorname{sp}}$ if and only if $f$ is a continuous function $X^{\operatorname{Alex}} \to Y$ .
The functors $F$ and $G$ are adjoint: $F \dashv G$ .
The composition $GF \colon \mathbf{PreOrd} \to \mathbf{PreOrd}$ is equal (not just isomorphic!) to the identity functor.
The restriction of $FG \colon \mathbf{Top} \to \mathbf{Top}$ to the category $\mathbf{Top}^{\operatorname{fin}}$ of finite topological spaces is equal to the identity functor.
If $Y$ is a topological space, then $Y$ is $T_0$ if and only if $Y^{\operatorname{sp}}$ is a poset.
If $(X,\leq)$ is a preorder, then $X$ is a poset if and only if $X^{\operatorname{Alex}}$ is $T_0$ .
The functors $F$ and $G$ give rise to adjoint equivalences
$\begin{align*}F\!:\mathbf{PreOrd}^{\operatorname{fin}} &\leftrightarrows \mathbf{Top}^{\operatorname{fin}}:\!G \\F\!:\mathbf{Pos}^{\operatorname{fin}} &\leftrightarrows \mathbf{Top}^{\operatorname{fin}}_{T_0}:\!G.\end{align*}$

Proof. (1) Suppose $f \colon X \to Y^{\operatorname{sp}}$ is monotone, let $Z \subseteq Y$ be a closed subset, and let $W = f^{-1}(Z)$ . Suppose $b \in W$ and $a \leq b$ . Since $f$ is monotone and $Z$ is closed, we get $f(a) \leq f(b)$ , i.e. $f(a) \in \overline{\{f(b)\}} \subseteq Z$ . We conclude that $a \in W$ , so $W$ is downward closed, hence closed in $X^{\operatorname{Alex}}$ .

Conversely, suppose $f \colon X^{\operatorname{Alex}} \to Y$ is continuous, and suppose $a \leq b$ in $X$ . Then $a \in \overline{\{b\}}$ , so by continuity we get $f(a) \in f\left(\overline{\{b\}}\right) \subseteq \overline{\{f(b)\}}$ , so $f(a) \leq f(b)$ .

(2) This is a restatement of (1): the map

$\begin{align*}\operatorname{Hom}_{\mathbf{PreOrd}}\big(X,G(Y)\big) &\stackrel\sim\to \operatorname{Hom}_{\mathbf{Top}}\big(F(X),Y\big) \\f &\mapsto f\end{align*}$

is a bijection.

(3) Since $\overline{\{x\}} = X_{\leq x}$ , we conclude that $y \in \overline{\{x\}}$ if and only if $y \leq x$ , so the specialisation preorder on $X^{\operatorname{Alex}}$ is the original preorder on $X$ .

(4) In general, the counit $FG(Y) \to Y$ is a continuous map on the same underlying space, so $FG(Y)$ is finer than $Y$ . Conversely, suppose $Z \subseteq FG(Y)$ is closed, i.e. $Z$ is a sieve for the specialisation preorder on $Y$ . This means that if $y \in Z$ , then $x \in \overline{\{y\}}$ implies $x \in Z$ ; in other words $\overline{\{y\}} \subseteq Z$ . If $Y$ and therefore $Z$ is finite, there are finitely many such $y$ , so $Z$ is the finite union

$Z = \bigcup_{y \in Z} \overline{\{y\}}$

of closed subsets of $Y$ . Thus any closed subset of $FG(Y)$ is closed in $Y$ , so the topologies agree.

(5) The relations $x \leq y$ and $y \leq x$ mean $x \in \overline{\{y\}}$ and $y \in \overline{\{x\}}$ . This is equivalent to the statement that a closed subset $Z \subseteq Y$ contains $x$ if and only if it contains $y$ . The result follows since a poset is a preorder where the first statement only happens if $x = y$ , and a $T_0$ space is a space where the second statement only happens if $x = y$ .

(6) Follows from (5) applied to $Y = F(X)$ since $X = G(Y)$ by (3).

(7) The equivalence $\mathbf{PreOrd}^{\operatorname{fin}} \leftrightarrows \mathbf{Top}^{\operatorname{fin}}$ follows from (3) and (4), and the equivalence $\mathbf{Pos}^{\operatorname{fin}} \leftrightarrows \mathbf{Top}^{\operatorname{fin}}_{T_0}$ then follows from (5) and (6). $\qedsymbol$

Example. The Alexandroff topology on the poset $[1] = \{0 \leq 1\}$ is the Sierpiński space $S = \{0,1\}$ with topology $\{\varnothing, \{1\}, S\}$ . As explained in this post, continuous maps $X \to S$ from a topological space $X$ to $S$ are in bijection with open subsets $U \subseteq X$ , where $f \colon X \to S$ is sent to $f^{-1}(1) \subseteq X$ (and $U \subseteq X$ to the indicator function $\mathbf 1_U \colon X \to S$ ).

Example. Let $X = \{x,y\}$ be a set with two elements. There are 4 possible topologies on $X$ , sitting in the following diagram (where vertical arrows indicate inclusion bottom to top):

${\arraycolsep=-1em\begin{array}{ccccc} & & \{\varnothing,\{x\},\{y\},X\} & & \\ & \ \ / & & \backslash\ \ & \\ \{\varnothing,\{x\},X\} & & & & \{\varnothing,\{y\},X\} \\ & \ \ \backslash & & /\ \ & \\ & & \{\varnothing,X\}.\! & & \end{array}}$

These correspond to 4 possible preorder relations $\{(a,b)\ |\ a \leq b\} \subseteq X\times X$ , sitting in the following diagram (where vertical arrows indicate inclusion top to bottom):

${\arraycolsep=-1.5em\begin{array}{ccccc} & & \{(x,x),(y,y)\} & & \\ & \ \ / & & \backslash\ \ & \\ \{(x,x),(x,y),(y,y)\} & & & & \{(x,x),(y,x),(y,y)\} \\ & \ \ \backslash & & /\ \ & \\ & & \{(x,x),(x,y),(y,x),(y,y)\}.\!\! & & \end{array}}$

We see that finer topologies (more opens) have stronger relations (fewer inequalities).

Example. The statement in (4) is false for infinite topological spaces. For instance, if $Y$ is the Zariski topology on a curve, then any set of closed points is downwards closed, but it is only closed if it’s finite. Or if $Y$ is a Hausdorff space, then the specialisation preorder is just the equality relation $\Delta_Y \subseteq Y \times Y$ , whose Alexandroff topology is the discrete topology.

I find the examples useful for remembering which way the adjunction goes: topological spaces generally have fewer opens than Alexandroff topologies on posets, so the continuous map should go $X^{\operatorname{Alex}} \to Y$ .

Remark. On any topological space $X$ , we can define the naive constructible topology as the topology with a base given by locally closed sets $U \cap Z$ for $U \subseteq X$ open and $Z \subseteq X$ closed. In the Alexandroff topology, a base for this topology is given by the locally closed sets $X_{\lessgtr x} := X_{\geq x} \cap X_{\leq x}$ : indeed these sets are clearly naive constructible, and any set of the form $S = U \cap Z$ for $U$ upward closed and $Z$ downward closed has the property $x \in S \Rightarrow X_{\lessgtr x} \subseteq S$ .

Thus, if $X$ is the Alexandroff topology on a preorder, we see that the naive constructible topology is discrete if and only if the preorder is a poset, i.e. if and only if $X$ is $T_0$ .

Lawvere–Tierney topologies (topologies 6/6)

Posted on 12 April 2022 by Remy

In the post of two weeks ago, we showed how Grothendieck topologies form a neat framework for the categorical aspects of the more familiar (at least to algebraic geometers) Grothendieck pretopologies. In this final post of the series, we take this one step further, to the notion of a Lawevere–Tierney topology on an arbitrary elementary topos $\mathscr E$ .

Definition. An elementary topos is a category $\mathscr E$ that has finite limits, is Cartesian closed, and has a subobject classifier (see last week’s post).

The only example we’re interested in today is the category $\mathscr E = [\mathscr C^{\operatorname{op}},\mathbf{Set}]$ of presheafs (of sets) on a small category $\mathscr C$ , which we saw last week has subobject classifiers. But in fact, any Grothendieck topos (sheaves of sets on a (small) site) is an example of an elementary topos, so one could even introduce further topologies on those.

Example. The category $\mathbf{Set}_{\text{fin}}$ of finite sets is an elementary topos, but not a Grothendieck topos since it is neither complete nor cocomplete.

Before giving the definition, we need to define one more structure on $\Omega$ : the meet. Recall that the intersection (or meet) of two monomorphisms $U \hookrightarrow X \hookleftarrow V$ is the fibre product

$U \wedge V := U \underset X\times V \hookrightarrow X.$

The intersection of $\mathbf 1 \times \Omega \hookrightarrow \Omega \times \Omega$ and $\Omega \times \mathbf 1 \hookrightarrow \Omega \times \Omega$ is the monomorphism $\mathbf 1 \hookrightarrow \Omega \times \Omega$ given by $(t,t)$ , which is classified by a map $\wedge \colon \Omega \times \Omega \to \Omega$ . Since $\mathbf 1 \to \Omega$ is the universal monomorphism, we see that $\mathbf 1 \hookrightarrow \Omega \times \Omega$ is the universal intersection of two subobjects, i.e. if $U \to X$ and $V \to X$ are classified by $f \colon X \to \Omega$ and $g \colon X \to \Omega$ respectively, then $U \wedge V$ is classified by the composition

$X \overset{(f,g)}\longrightarrow \Omega \times \Omega \overset\wedge\longrightarrow \Omega.$

(If we denote this simply by $f \wedge g \colon X \to \Omega$ , then $\wedge \colon \Omega \times \Omega \to \Omega$ is $\operatorname{pr}_1 \wedge \operatorname{pr}_2$ .)

Definition. Let $\mathscr E$ be an elementary topos with subobject classifier $t \colon \mathbf 1 \to \Omega$ . Then a Lawvere–Tierney topology on $\mathscr E$ is a morphism $j \colon \Omega \to \Omega$ such that the following diagrams commute:

$\begin{array}{ccc}\mathbf 1\!\! & \stackrel t\to\!\! & \!\!\!\Omega \\ & \!\!\underset{t\!\!\!}{}\searrow\!\! & \downarrow j\!\! \\[-.2em] & & \!\!\!\Omega,\!\!\end{array}\qquad\qquad\begin{array}{ccc}\Omega\!\! & \stackrel j\to\!\! & \!\!\!\Omega \\ & \!\!\underset{j\!\!\!}{}\searrow\!\! & \downarrow j\!\! \\[-.3em] & & \!\!\!\Omega,\!\!\end{array}\qquad\qquad\quad\begin{array}{ccc}\Omega \times \Omega\!\!\! & \stackrel\wedge\to\!\! & \!\!\!\Omega \\ \!\!\!\!\!\!\!\!\!\!\!\!j\times j \downarrow & & \downarrow j\!\! \\ \Omega \times \Omega\!\!\! &\stackrel\wedge\to\!\! & \!\!\!\Omega.\!\!\end{array}$

We saw two weeks ago that a Grothendieck topology is a certain subpresheaf $J \subseteq \mathbf{Siv}$ , and last week that $\mathbf{Siv}$ is a subobject classifier $\Omega$ on $[\mathscr C^{\operatorname{op}},\mathbf{Set}]$ . Thus a subpresheaf $J \subseteq \Omega$ is classified by a morphism $j \colon \Omega \to \Omega$ , which we saw last week is given by $S \mapsto (S \in J)$ .

Lemma. The subpresheaf $J \subseteq \Omega$ is a Grothendieck topology on $\mathscr C$ if and only if $j \colon \Omega \to \Omega$ is a Lawvere–Tierney topology on $[\mathscr C^{\operatorname{op}},\mathbf{Set}]$ . In particular, Grothendieck topologies on $\mathscr C$ are in bijective correspondence with Lawvere–Tierney topologies on $[\mathscr C^{\operatorname{op}},\mathbf{Set}]$ .

Thus Lawvere–Tierney topologies are an internalisation of the notion of Grothendieck topology to an arbitrary elementary topos $\mathscr E$ .

Proof of Lemma. By definition of the morphism $j$ , we have a pullback square

$\begin{array}{ccc}J & \to & \mathbf 1 \\ \downarrow & & \downarrow \\ \Omega & \stackrel j\to & \Omega.\!\end{array}$

The first commutative diagram in the definition above means that the top arrow has a section $\mathbf 1 \to J$ such that the composition $\mathbf 1 \to J \hookrightarrow \Omega$ is $t$ , i.e. $\mathbf 1 \subseteq J$ as subobjects of $\Omega$ . Since $t \colon \mathbf 1 \to \Omega$ is the map taking $1 \in \mathbf 1(X)$ to the maximal sieve $h_X \subseteq h_X$ for any $X \in \mathscr C$ , this means exactly that $h_X \in J(X)$ for all $X \in \mathscr C$ , which is condition 1 of a Grothendieck topology. For the second, consider the pullback

$\begin{array}{ccccc}J' & \to & J & \to & \mathbf 1 \\ \downarrow & & \downarrow & & \downarrow \\ \Omega & \stackrel j\to & \Omega & \stackrel j\to & \Omega.\!\end{array}$

The condition $jj=j$ means that $J' \cong J$ as subobjects of $\Omega$ . We already saw that $\mathbf 1 \subseteq J$ for a Grothendieck or Lawvere–Tierney topology, so pulling back along $j$ gives $J \subseteq J'$ . Thus the second diagram in the definition of a Lawvere–Tierney topology commutes if and only if $J' \subseteq J$ , i.e. if $S \in \Omega(X) = \mathbf{Siv}(X)$ with $j_X(S) \in J(X)$ , then $S \in J(X)$ . But $j_X \colon \Omega(X) \to \Omega(X)$ is given by $S \mapsto (S \in J)$ , so this is exactly axiom 3 of a Grothendieck topology.

For the third diagram, we first claim that $j_X \colon \Omega(X) \to \Omega(X)$ is monotone for all $X \in \mathscr C$ if and only if $J$ satisfies axiom 2 of a Grothendieck topology. Indeed, if $j$ is monotone and $S, S' \in \Omega(X)$ satisfy $S \subseteq S'$ and $S \in J(X)$ , then the inclusion $h_X = (S \in J) \subseteq (S' \in J)$ shows $(S' \in J) = h_X$ , so $S' \in J(X)$ by axiom 3. Conversely, if $J$ satisfies axiom 2 and $S,S' \in \Omega(X)$ satisfy $S \subseteq S'$ , then for any $f \colon Y \to X$ we have $f^*S \subseteq f^*S'$ , so $f^*S \in J(Y) \Rightarrow f^*S' \in J(Y)$ , i.e. $(S \in J) \subseteq (S' \in J)$ .

The third diagram in the definition above says that the map $j_X \colon \Omega(X) \to \Omega(X)$ given by $S \mapsto (S \in J)$ is a morphism of meet semilattices. This implies in particular that $j_X$ is monotone, as $S \subseteq S'$ if and only if $S \wedge S' = S$ , so the third diagram above implies axiom 2 of a Grothendieck topology.

Conversely, if $J$ is a Grothendieck topology, then axiom 2 implies that $j_X \colon \Omega \to \Omega$ is monotone. In particular, $j_X(S \cap T) \subseteq j_X(S) \cap j_X(T)$ for any $S, T \in \Omega(X)$ , since $S \cap T \subseteq S, T$ . For the reverse implication, if $f \colon Y \to X$ satisfies $f \in (S \in J)(Y) \cap (T \in J)(Y)$ , then $f^*S \in J(Y)$ and $f^*T \in J(Y)$ , so the remark of two weeks ago shows that $f^*(S \cap T) \in J(Y)$ , i.e. $f \in ((S \cap T) \in J)(Y)$ . We see that $j_X(S \cap T) = j_X(S) \cap j_X(T)$ , showing that $j_X$ is a morphism of meet semilattices. $\qedsymbol$

Subobject classifiers on presheaf categories (topologies 5/6)

Posted on 3 April 2022 by Remy

In the first post of this series, we saw how subobjects of representable presheaves $h_U \colon \mathscr C^{\operatorname{op}} \to \mathbf{Set}$ correspond to sieves on $U$ . Last week, we saw how sieves give a convenient language for defining Grothendieck topologies on a small category. In next week’s (hopefully) final instalment of this series, we will generalise this even further to Lawvere–Tierney topologies on an arbitrary topos. Today’s post defines the last object we need to do this, which we will show generalises the presheaf $\mathbf{Siv}$ from last week:

Definition. Let $\mathscr C$ be a (possibly large) category with a terminal object $\mathbf{1}$ . Then a subobject classifier on $\mathscr C$ is a monomorphism $\mathbf{1} \to \Omega$ in $\mathscr C$ such that for every monomorphism $U \to X$ in $\mathscr C$ , there exists a unique arrow $X \to \Omega$ such that there is a pullback diagram

$\begin{array}{ccc}U & \to & \mathbf{1} \\ \downarrow & & \downarrow \\ X & \to & \Omega.\!\end{array}$

That is, $\mathbf{1} \to \Omega$ is the “universal” monomorphism in $\mathscr C$ , i.e. the pair $(\Omega,\mathbf{1} \hookrightarrow \Omega)$ represents the (possibly large) presheaf $X \mapsto \{\text{monomorphisms } U \hookrightarrow X\}/\cong$ , where $\cong$ denotes isomorphism in the slice category $\mathscr C/X$ . It is an easy exercise to show that any representative $(\Omega, T \hookrightarrow \Omega)$ of this presheaf actually has the form described above, i.e. $T$ is a terminal object (apply the uniqueness property above to the identity monomorphism $X \hookrightarrow X$ , and use the pullback square

$\begin{array}{ccc}T & = & T \\ \shortparallel & & \downarrow \\ T & \to & \Omega\end{array}$

coming from the hypothesis that $T \hookrightarrow \Omega$ is a monomorphism).

Example. If $\mathscr C = \mathbf{Set}$ , then the two-point set $\Omega = \{0,1\}$ with its natural inclusion $\mathbf 1 \to \Omega$ given by $1 \mapsto 1$ is a subobject classifier: the monomorphism $U \subseteq X$ corresponds to the indicator function $\delta_U \colon X \to \Omega$ that is $1$ on $U$ and $0$ on its complement. (In other situations I would denote this by $\mathbf 1_U$ , but that notation was already used in this series to denote the representable presheaf $h_U$ .)

It’s even more natural to take $\Omega$ to be the power set $\{\varnothing,\mathbf 1\}$ of $\mathbf{1}$ . As in the first post of this series, we think of $\mathbf 1$ representing “true” and $\varnothing$ representing “false”. The generalisation of the power set of $\mathbf{1}$ to presheaf categories is the presheaf $\mathbf{Siv}$ of subpresheaves of $h_X$ defined last week:

Lemma. Let $\mathscr C$ be a small category. Then the presheaf $\mathbf{Siv}$ together with the map $\mathbf 1 \to \mathbf{Siv}$ taking the unique section $1 \in \mathbf 1(X)$ to the maximal sieve $h_X \subseteq h_X$ for any $X \in \mathscr C$ is a subobject classifier in $[\mathscr C^{\operatorname{op}},\mathbf{Set}]$ .

Proof. Note that the prescribed map $\mathbf 1 \to \mathbf{Siv}$ is a morphism of presheaves, since the inverse image of the maximal sieve $h_X$ under any morphism $f \colon Y \to X$ in $\mathscr C$ is the maximal sieve $h_Y$ . Again using the notation from last week, if $\mathscr G \hookrightarrow \mathscr F$ is any monomorphism of presheaves, we get a morphism of presheaves $\phi \colon \mathscr F \to \Omega$ defined on $X \in \mathscr C$ by

$\begin{align*}\mathscr F(X) &\to \mathbf{Siv}(X) \\s &\mapsto (s \in \mathscr G).\end{align*}$

If $f \colon Y \to X$ is a morphism in $\mathscr C$ , then for any $Z \in \mathscr C$ we have

$\begin{align*}\big(f^*(s \in \mathscr G)\big)(Z) &= \{g \colon Z \to Y\ |\ fg \in (s \in \mathscr G)(Z)\} \\&= \{g \colon Z \to Y\ |\ (fg)^*(s) \in \mathscr G(Z)\} \\&= \{g \colon Z \to Y\ |\ g^*(f^*(s)) \in \mathscr G(Z)\} = (f^*s \in \mathscr G)(Z),\end{align*}$

showing that $f^*\phi(s) = \phi(f^*s)$ , so $\phi$ is indeed a natural transformation. We already noted last week that $(s \in \mathscr G) = h_X$ for $s \in \mathscr F(X)$ if and only if $s \in \mathscr G(X)$ , so $\mathscr G$ is the pullback

$\begin{array}{ccc}\mathscr G & \to & \mathbf{1} \\ \downarrow & & \downarrow \\ \mathscr F & \to & \mathbf{Siv}.\!\end{array}$

Conversely, if $\psi \colon \mathscr F \to \mathbf{Siv}$ is any morphism with this property and $s \in \mathscr F(X)$ , then $s \in \mathscr G(X)$ if and only if $\psi(s) = h_X$ , which together with naturality of $\psi$ gives

$\begin{align*}(s \in \mathscr G)(Y) &= \{f \colon Y \to X\ |\ f^*s \in \mathscr G(Y)\} \\&= \{f \colon Y \to X\ |\ \psi(f^*s) = h_Y\} \\&= \{f \colon Y \to X\ |\ f^*\psi(s) = h_Y\} \\&= \left\{f \colon Y \to X\ |\ \operatorname{id}_Y \in \big(f^*\psi(s)\big)(Y)\right\} \\&= \{f \colon Y \to X\ |\ f \circ \operatorname{id}_Y \in (\psi(s))(Y)\} = (\psi(s))(Y),\end{align*}$

so $\psi(s) = (s \in \mathscr G)$ . $\qedsymbol$

We will discuss some other properties of subobject classifiers in future posts.

Grothendieck topologies (topologies 4/6)

Posted on 28 March 2022 by Remy

This post is the first goal in a series on sieves (subobjects of representable presheaves); I will give another generalisation in the next two posts. In the first post of the series, I defined sieves and gave basic examples, and last week I showed how the sheaf condition on a site can be stated in terms of sieves:

Corollary. Let $\mathscr C$ be a (small) site. For a set of morphisms $\mathscr U = \{U_i \to U\}_{i \in I}$ with the same target, write $S_{\mathscr U} \subseteq h_U$ for the presheaf image of $\coprod_{i\in I} h_{U_i} \to h_U$ . Then a presheaf $\mathscr F \colon \mathscr C^{\operatorname{op}} \to \mathbf{Set}$ is a sheaf if and only if for every covering $\mathscr U = \{U_i \to U\}_{i \in I}$ in $\mathscr C$ , the inclusion $S_{\mathscr U} \hookrightarrow h_U$ induces an isomorphism

$\operatorname{Hom}(h_U,\mathscr F) \stackrel\sim\to \operatorname{Hom}(S_{\mathscr U},\mathscr F).$

Thus, if $\mathscr C$ is a site (a small category with a Grothendieck pretopology), we should be able to obtain the category $\mathbf{Sh}(\mathscr C) \subseteq \mathbf{PSh}(\mathscr C)$ of sheaves purely in terms of sieves. This is the notion of a Grothendieck topology that we describe at the end of this post.

Before giving the definition, note that any morphism $f \colon Y \to X$ in $\mathscr C$ gives a pullback $\mathbf{Siv}(X) \to \mathbf{Siv}(Y)$ taking $S \subseteq h_X$ to its inverse image under $h_f \colon h_Y \to h_X$ (I avoid the word ‘pullback’ here to make sure this is truly a subpresheaf and not a presheaf with a monomorphism to $h_Y$ defined uniquely up to unique isomorphism). Thus, $\mathbf{Siv}$ is itself a presheaf $\mathscr C^{\operatorname{op}} \to \mathbf{Set}$ (it takes values in $\mathbf{Set}$ since $\mathscr C$ is small).

Also note the following method for producing sieves: if $\mathscr F$ is a presheaf, $\mathscr G \subseteq \mathscr F$ a subpresheaf, and $s \in \mathscr F(X)$ a section over some $X \in \mathscr C$ , we get a sieve $(s \in \mathscr G) \in \mathbf{Siv}(X)$ by

$(s \in \mathscr G)(Y) = \left\{f \colon Y \to X\ \big|\ f^*(s) \in \mathscr G(Y)\right\}.$

By the Yoneda lemma, this is just the inverse image of $\mathscr G \subseteq \mathscr F$ along the morphism $h_X \to \mathscr F$ classifying $s$ . Note that $(s \in \mathscr G)$ is the maximal sieve $h_X$ if and only if $s \in \mathscr G(X)$ .

Definition. Let $\mathscr C$ be a small category. Then a Grothendieck topology on $\mathscr C$ consists of a subpresheaf $J \subseteq \mathbf{Siv}$ such that

For all $X \in \mathscr C$ , the maximal sieve $h_X \subseteq h_X$ is in $J(X)$ .
If $S \in J(X)$ and $S' \in \mathbf{Siv}(X)$ with $S \subseteq S'$ , then $S' \in J(X)$ .
If $S \in \mathbf{Siv}(X)$ is a sieve such that $(S \in J) \in J(X)$ , then $S \in J(X)$ (equivalently, then $(S \in J)$ is the maximal sieve $h_X$ ).

The sieves $S \in J(X)$ are called covering sieves. Since $J$ is a presheaf, we see that for any $f \colon Y \to X$ and any covering sieve $S \subseteq h_X$ , the pullback $f^*S \subseteq h_Y$ is covering. Condition 2 says that any sieve containing a covering sieve is covering. In the presence of condition 1, conditions 2 and 3 together are equivalent to the local character found in SGA IV $_1$ , Exp. II, Def. 1.1:

If $S, S' \in \mathbf{Siv}(X)$ with $S \in J(X)$ , such that for every morphism $h_Y \to S$ the inverse image of $S' \subseteq h_X$ along $h_Y \to S \to h_X$ is in $J(Y)$ , then $S' \in J(X)$ .

Indeed, applying this criterion when $S \subseteq S'$ immedately shows $S' \in J(X)$ if $S \in J(X)$ , since the inverse image of $S' \subseteq h_X$ along $h_Y \to S \to h_X$ is the maximal sieve $h_Y$ . Thus the local character implies criterion 2. The local character says that if $(S' \in J)$ contains a covering sieve $S$ , then $S'$ is covering. Assuming criterion 2, the sieve $(S' \in J)$ contains a covering sieve if and only if $(S' \in J)$ is itself covering, so the local character is equivalent to criterion 3.

Remark. One property that follows from the axioms is that $J(X)$ is closed under binary intersection, i.e. if $S, T \in J(X)$ then $(S \cap T) \in J(X)$ . Indeed, if $f \in S(Y)$ for some $f \colon Y \to X$ , then

$f^*(S \cap T) = f^*S \cap f^*T = h_Y \cap f^*T = f^*T \in J(Y),$

so $S \subseteq ((S \cap T) \in J)$ . Axioms 2 and 3 give $(S \cap T) \in J(X)$ .

Example. Let $\mathcal Cov(\mathscr C)$ be a pretopology on the (small) category $\mathscr C$ ; see Tag 00VH for a list of axioms. For each $X \in \mathscr C$ , define the subset $J(X) \subseteq \mathbf{Siv}(X)$ as those $S \subseteq h_X$ that contain a sieve of the form $S_{\mathscr U}$ for some covering $\mathscr U = \{U_i \to X\}$ in $\mathcal Cov(\mathscr C)$ . (See the corollary at the top for the definition of $S_{\mathscr U}$ .) Concretely, this means that there exists a covering $\{f_i \colon U_i \to X\}_{i \in I} \in \mathcal Cov(\mathscr C)$ such that $f_i \in S(U_i)$ for all $i \in I$ , i.e. $X$ is covered by morphisms $f_i \colon U_i \to X$ that are in the given sieve $S$ .

Lemma. The association $X \mapsto J(X)$ is a topology. It is the coarsest topology on $\mathscr C$ for which each $S_{\mathscr U}$ for $\mathscr U \in \mathcal Cov(\mathscr C)$ is a covering sieve.

Proof. We will use the criteria of Tag 00VH. If $S \in J(X)$ , then there exists $\mathscr U = \{U_i \to X\}_{i \in I} \in \mathcal Cov(\mathscr C)$ with $S_{\mathscr U} \subseteq S$ . If $f \colon Y \to X$ is any morphism in $\mathscr C$ , then $f^*\mathscr U = \{U_i \times_X Y \to Y\}_{i \in I} \in \mathcal Cov(\mathscr C)$ by criterion 3 of Tag 00VH. But $S_{f^*\mathscr U} = f^*S_{\mathscr U}$ , because a morphism $g \colon U \to Y$ factors through $U_i \times_X Y$ if and only if $fg \colon U \to X$ factors through $U_i$ . Thus, $S_{f^*\mathscr U} = f^*S_{\mathscr U} \subseteq f^*S$ , so $f^*S \in J(Y)$ , and $J$ is a subpresheaf of $\mathbf{Siv}$ .

Condition 1 follows immediately from criterion 1 in Tag 00VH, and condition 2 is satisfied by definition. For condition 3, suppose $S \in \mathbf{Siv}(X)$ satisfies $(S \in J) \in J(X)$ . Then there exists $\mathscr U = \{f_i \colon U_i \to X\}_{i \in I} \in \mathcal Cov(\mathscr C)$ with $S_{\mathscr U} \subseteq (S \in J)$ . This means that $f_i \in (S \in J)(U_i)$ for all $i$ , i.e. $f_i^*S \in J(U_i)$ for all $i$ . Thus, for each $i \in I$ there exists $\mathscr V_i = \{g_{ij} \colon V_{ij} \to U_i\}_{j \in J_i}$ in $\mathcal Cov(\mathscr C)$ such that $S_{\mathscr V_i} \subseteq f_i^*S$ , i.e. $f_ig_{ij} \in S(X)$ for all $i \in I$ and all $j \in J_i$ . Thus, if $\mathscr V$ denotes $\{f_ig_{ij} \colon V_{ij} \to X\}_{i \in I, j \in J_i}$ , then we get $S_{\mathscr V} \subseteq S$ . But $\mathscr V$ is a covering by criterion 2 of Tag 00VH, so $S \in J(X)$ .

If $J'$ is any other Grothendieck topology for which each $S_{\mathscr U}$ for $\mathscr U \in \mathcal Cov(\mathscr C)$ is covering, then $J'$ contains $J$ by criterion 2. $\qedsymbol$

To state the obvious (hopefully), the notion of sheaf can therefore be defined on a Grothendieck topology in a way that coincides with the usual notion for a Grothendieck pretopology:

Definition. Let $\mathscr C$ be a small category, and let $J \subseteq \mathbf{Siv}$ be a Grothendieck topology. Then a presheaf $\mathscr F \colon \mathscr C^{\operatorname{op}} \to \mathbf{Set}$ is a sheaf if for any $X \in \mathscr C$ and any $S \in J(X)$ , the map $S \hookrightarrow h_X$ induces an isomorphism

$\operatorname{Hom}(h_X,\mathscr F) \stackrel\sim\to \operatorname{Hom}(S,\mathscr F).$

Thus, a Grothendieck topology is an internal characterisation (inside $\mathbf{PSh}(\mathscr C)$ ) of which morphisms $S \to h_X$ one needs to localise to get $\mathbf{Sh}(\mathscr C,J)$ . In the last two posts, we will generalise this even further to a Lawvere–Tierney topology on an arbitrary topos.

Covering sieves and the sheaf condition (topologies 3/6)

Posted on 20 March 2022 by Remy

In the first post of this series, I explained how subobjects of the constant presheaf (resp. constant sheaf) $\mathbf 1_X$ on a small category (resp. small site) with terminal object $X$ correspond to left closed (resp. local) properties on $\mathscr C$ . In this post, I will explain the main examples that intervene in setting up topoi, and show how to define the sheaf condition using sieves (instead of coverings).

For simplicity, assume $\mathscr C$ is a small category with fibre products.

Definition. Given a set of morphisms $\mathscr U = \{f_i \colon U_i \to U\}_{i \in I}$ with the same target $U \in \mathscr C$ , define the sieve $S_{\mathscr U} \subseteq h_U$ generated by $\mathscr U$ as the sieve on $U$ of those morphisms $V \to U$ that factor through some $f_i \colon U_i \to U$ .

It is in a sense the right ideal in $\operatorname{Hom}(-,U)$ generated by the $f_i$ . What does this look like as a subobject of $h_U$ ?

Example. If $I$ has one element, i.e. $\mathscr U = \{V \to U\}$ , then $S_{\mathscr U}$ is the image of the morphism of representable presheaves $h_V \to h_U$ . In the case where $V \to U$ is already a monomorphism (this is always the case when $\mathscr C$ is a poset, such as $\operatorname{Open}(X)$ for some topological space $X$ ), then $h_V \to h_U$ is itself injective (this is the definition of a monomorphism!), so $S_{\mathscr U}$ is just $h_V$ .

In general, $S_{\mathscr U}$ is the image of the map

$\coprod_{i \in I} h_{U_i} \to h_U$

induced by the maps $U_i \to U$ . Indeed, an element of $h_U(V)$ is a morphism $f \colon V \to U$ , and it comes from some $h_{U_i}(V)$ if and only if $f$ factors through $f_i \colon U_i \to U$ .

This shows that, in fact, every sieve $S \subseteq h_X$ is of this form for some set $\{U_i \to U\}_{i \in I}$ : take as index set (the objects of) the slice category $(h \downarrow S)$ , which as in the previous post gives a surjection $\coprod_{(V,\alpha)} h_V \to S$ . This corresponds to generating an ideal by all its elements.

But we can also characterise $S_{\mathscr U}$ without using the word ‘image’ (which somehow computes its first syzygy):

Lemma. Let $\mathscr U = \{U_i \to U\}$ be a set of morphisms with common target, and $S_{\mathscr U}$ the sieve generated by $\mathscr U$ . Then $S_{\mathscr U}$ is the coequaliser of the diagram

$\coprod_{i,j \in I} h_{U_i \underset U\times U_j} \rightrightarrows \coprod_{i \in I} h_{U_i},$

where the maps are induced by the two projections $I^2 \to I$ .

We will give two proofs, one using the description of coequalisers of sets, and the other using that presheaves are colimits of representable presheaves, as discussed in the previous post.

Proof 1. The diagram

$\begin{array}{ccc}\displaystyle\coprod_{i,j \in I} h_{U_i \underset U\times U_j} & \to & \displaystyle\coprod_{i \in I} h_{U_i} \\ \downarrow & & \downarrow \\ \displaystyle\coprod_{j \in I} h_{U_j} & \to & h_U \end{array}$

is a pullback, by the universal property of fibre products $U_i \times_U U_j$ and since fibre products with a fixed set/presheaf of sets commute with coproducts. Then the same goes for the square

since $S_{\mathscr U} \to h_U$ is a monomorphism. But $\coprod_{i \in I} h_{U_i} \to S_{\mathscr U}$ is an epimorphism (objectwise surjection) by definition, so this square is a pushout as well (in $\mathbf{Set}$ , epimorphisms are regular). $\qedsymbol$

Proof 2. By the previous post, the presheaf $S_{\mathscr U}$ is the colimit over $(V,\alpha) \in (h \downarrow S_{\mathscr U})$ of $h_V$ (see post for precise statement). Let $D \colon (\bullet \rightrightarrows \bullet) \to \mathbf{Set}$ be the diagram $I^2 \rightrightarrows I$ of the two projections, and let $\mathcal I = \bigcup D = (h \downarrow D)^{\operatorname{op}}$ be the category of elements of $D$ , as in this post. There is a natural functor $F \colon \mathcal I \to (h \downarrow S_{\mathscr U})$ taking $(i,j) \in I^2$ to $(U_i \times_U U_j,h_{U_i \times_U U_j} \to S_{\mathscr U})$ and $i \in I$ to $(U_i,h_{U_i} \to S_{\mathscr U})$ , taking the morphisms $i \leftarrow (i,j) \to j$ in $\mathcal I$ to the projections $U_i \leftarrow U_i \times_U U_j \to U_j$ . We claim that $F$ is cofinal, hence the colimit can be computed over $\mathcal I$ instead (see Tag 04E7).

To verify this, we use the criteria of Tag 04E6. If $(V,\alpha) \in (h \downarrow S_{\mathscr U})$ , then by definition the composition $h_V \stackrel\alpha\to S_{\mathscr U} \hookrightarrow h_U$ is given by a morphism $f \colon V \to U$ that is contained in $S_{\mathscr U}(V)$ . Since $S_{\mathscr U}$ is generated by the $U_i$ , this factors through some $V \to U_i$ over $S_{\mathscr U}$ , giving a map $(V,\alpha) \to F(i)$ .

If $(V,\alpha) \to F(i)$ and $(V,\alpha) \to F(j)$ are two such maps, they factor uniquely through $(V,\alpha) \to F(i,j)$ . The general result for $(V,\alpha) \to F(x)$ and $(V,\alpha) \to F(y)$ for $x,y \in \mathcal I$ (either of the form $i$ or of the form $(i,j)$ ) follows since elements of the form $(i,j)$ always map to the elements $i$ and $j$ , showing that the category $((V,\alpha) \downarrow F)$ is weakly connected. $\qedsymbol$

Corollary. Let $S_{\mathscr U}$ as above, and let $\mathscr F$ be a presheaf on $\mathscr C$ . Then

$\operatorname{Hom}(S_{\mathscr U},\mathscr F) \stackrel\sim\to \operatorname{Eq}\left( \prod_{i \in I} \mathscr F(U_i) \rightrightarrows \prod_{i,j\in I} \mathscr F\Big(U_i \underset U\times U_j\Big) \right).$

Proof. By the lemma above, we compute

$\begin{align*}\operatorname{Hom}(S_{\mathscr U},\mathscr F) &\cong \operatorname{Hom}\left(\operatorname{Coeq}\left(\coprod_{i \in I} h_{U_i \underset U \times U_j} \rightrightarrows \coprod_{i \in I} h_{U_i}\right), \mathscr F\right) \\&\cong \operatorname{Eq}\left(\prod_{i \in I} \operatorname{Hom}(h_{U_i},\mathscr F) \rightrightarrows \operatorname{Hom}\Big(h_{U_i \underset U\times U_j},\mathscr F\Big)\right),\end{align*}$

so the result follows from the Yoneda lemma. $\qedsymbol$

Corollary. Let $\mathscr C$ be a (small) site. Then a presheaf $\mathscr F \colon \mathscr C^{\operatorname{op}} \to \mathbf{Set}$ is a sheaf if and only if for every object $U \in \mathscr C$ and every covering $\{U_i \to U\}_{i \in I}$ in the site, the inclusion $S_{\mathscr U} \to h_U$ induces an isomorphism

$\operatorname{Hom}(h_U,\mathscr F) \stackrel\sim\to \operatorname{Hom}(S_{\mathscr U},\mathscr F).$

Proof. Immediate from the previous corollary. $\qedsymbol$

Thus, the category of sheaves on $\mathscr C$ can be recovered from $[\mathscr C^{\operatorname{op}},\mathbf{Set}]$ if we know at which subobjects $S \subseteq h_U$ we should localise (make the inclusion invertible). Next week, we will use this to give a definition of a Grothendieck topology, abstracting and generalising the notion of a site (i.e. Grothendieck pretopology).

Presheaves are colimits of representables (topologies 2/6)

Posted on 11 March 2022 by Remy

Last week, I started a series on sieves and Grothendieck topoi. This is a short intermezzo on a well-known lemma from category theory that I will need for next week’s instalment.

Let $\mathscr C$ be a small category. Recall that a presheaf on $\mathscr C$ is a functor $\mathscr C^{\operatorname{op}} \to \mathbf{Set}$ . Examples include the representable presheaves $h_X$ for $X \in \mathscr C$ , given by $\operatorname{Hom}(-,X)$ . The Yoneda lemma says that for any presheaf $F \colon \mathscr C^{\operatorname{op}} \to \mathbf{Set}$ and any $X \in \mathscr C$ , the map

$\begin{align*}\operatorname{Nat}(h_X,F) &\to F(X) \\\alpha &\mapsto \alpha_X(\operatorname{id}_X)\end{align*}$

is an isomorphism. Applying this to $F = h_Y$ shows that the Yoneda embedding

$\begin{align*}h \colon \mathscr C &\to [\mathscr C^{\operatorname{op}},\mathbf{Set}] \\X &\mapsto h_X\end{align*}$

is fully faithful.

Given a functor $F \colon \mathscr C^{\operatorname{op}} \to \mathbf{Set}$ , write $(h \downarrow F)$ for the comma category whose objects are pairs $(X,\alpha)$ where $X \in \mathscr C$ and $\alpha \colon h_X \to F$ is a morphism (natural transformation) in $[\mathscr C^{\operatorname{op}},\mathbf{Set}]$ . A morphism $f \colon (X,\alpha) \to (Y,\beta)$ is a morphism $f \colon X \to Y$ such that the triangle

(1) $\begin{array}{ccccc}\!\!h_X\!\!\!\!\! & & \!\!\!\!\!\stackrel{h_f}\longrightarrow\!\!\!\!\! & & \!\!\!\!\!h_Y\!\! \\ & \!\!\!\!{\underset{\alpha}{}}\!\!\searrow\!\!\!\! & & \!\!\!\!\swarrow\!\!{\underset{\beta}{}}\!\!\!\! & \\[-.3em] & & F\! & & \end{array}$

of natural transformations commutes, where $h_f \colon \operatorname{Hom}(-,X) \to \operatorname{Hom}(-,Y)$ denotes postcomposition by $f$ . Note that $(h \downarrow F)$ is again a small category, and there is a forgetful functor $U \colon (h \downarrow F) \to \mathscr C$ taking $(X,\alpha)$ to $X \in \mathscr C$ .

By the Yoneda lemma, the category $(h \downarrow F)$ is isomorphic (not just equivalent!) to the category $\int F$ of pairs $(X,s)$ with $X \in \mathscr C$ and $s \in F(X)$ with morphisms $f \colon (X,s) \to (Y,t)$ given by morphisms $f \colon X \to Y$ in $\mathscr C$ such that $F(f)(t) = s$ . It’s convenient to keep both points of view.

Lemma. Let $\mathscr C$ be a small category, and let $F \colon \mathscr C^{\operatorname{op}} \to \mathbf{Set}$ be a functor.

The object $F \in [\mathscr C^{\operatorname{op}},\mathbf{Set}]$ is naturally a cocone under $hU \colon (h \downarrow F) \to [\mathscr C^{\operatorname{op}},\mathbf{Set}]$ via the morphisms $\alpha \colon h_X \to F$ .
This cocone makes $F$ the colimit of the diagram $hU$ of representable functors.

In particular, any presheaf on a small category is a colimit of representable presheaves.

Proof. A cocone under $hU$ is a presheaf $G$ with a natural transformation $\phi \colon hU \to G$ to the constant diagram $(h \downarrow F) \to [\mathscr C^{\operatorname{op}},\mathbf{Set}]$ with value $G$ . This means every $(X,\alpha) \in (h \downarrow F)$ is taken to a natural transformation $\phi_{(X,\alpha)} \colon h_X \to G$ , such that for any morphism $f \colon (X,\alpha) \to (Y,\beta)$ , the square

$\begin{array}{ccc} h_X & \stackrel{\phi_{(X,\alpha)}}\longrightarrow & G \\ \!\!\!\!\!\!\!\!\!{h_f}\downarrow & & |\!| \\ h_Y & \underset{\phi_{(Y,\beta)}}\longrightarrow & G\end{array}$

commutes. By the Yoneda lemma, such a datum corresponds to an association of elements $\phi_{(X,s)} \in G(X)$ for all $(X,s) \in \int F$ such that for every $f \colon (X,s) \to (Y,t)$ with $F(f)(t) = s$ , we have $G(f)(\phi_{(Y,t)}) = \phi_{(X,s)}$ .

(1) To make $F$ a cocone under $hU$ , simply take $\phi_{(X,s)} = s \in F(X)$ .

(2) Given any other cocone $\phi \colon hU \to G$ under $hU$ , define the natural transformation $\eta \colon F \to G$ by

$\begin{align*}\eta_X \colon F(X) &\to G(X) \\s &\mapsto \phi_{(X,s)}.\end{align*}$

Naturality follows since $F(f)(t) = s$ implies $G(f)(\phi_{(Y,t)}) = \phi_{(X,s)}$ . It is clear that $\eta$ is the unique natural transformation of cocones $F \to G$ under $hU$ , showing that $F$ is the colimit. $\qedsymbol$

One can also easily rewrite this argument in terms of natural transformations $h_X \to G$ . For instance, the universal cocone $hU \to F$ is the natural transformation $\phi \colon hU \to F$ of functors $(h \downarrow F) \to [\mathscr C^{\operatorname{op}},\mathbf{Set}]$ given on $(X,\alpha) \in (h \downarrow F)$ by $\alpha \colon h_X \to F$ . Naturality of $\phi$ follows at once from (1). But checking that this thing is universal is a bit more tedious in this language.

Example. A standard example where this point of view is useful is simplicial sets. Let $\Delta$ be the category of finite nonempty totally ordered sets, with (weakly) monotone increasing functions as morphisms. A simplicial set is a functor $\Delta^{\operatorname{op}} \to \mathbf{Set}$ , and we often think of them as combinatorial models for topological spaces. The representable ones are the standard $n$ -simplices $\Delta^n = \operatorname{Hom}(-,[n])$ , where $[n]$ is the totally ordered set $\{0,\ldots,n\}$ for $n \in \mathbf Z_{\geq 0}$ .

If $X \colon \Delta^{\operatorname{op}} \to \mathbf{Set}$ is a simplicial set, its value at $[n]$ is called the $n$ -simplices $X_n$ of $X$ . By the Yoneda lemma, this is $\operatorname{Hom}(\Delta^n,X)$ . Then the story above is saying that a simplicial set is the colimit over all its $n$ -simplices for $n \in \mathbf Z_{\geq 0}$ . This is extremely useful, as many arguments proceed by attaching simplices one at a time.

Lovely little lemmas

Or maybe ‘amusing observations’